3 autograph letters signed to Archibald Smith plus 4-page mathematical autograph manuscript
Publisher Information: Cambridge: 1863-68.
Three Mathematical Autograph Letters, Signed, Plus a Four Page Autograph Mathematics Manuscript
Cayley, Arthur (1821-95). (1) Autograph letter signed (“A. C.”) to Archibald Smith (1813-72), written in the margins of an autograph letter signed from Smith to Cayley. 1 page. London, 9 August 1863 [date of Smith’s letter]. 280 x 226 mm. (2) Autograph letter signed to Smith. 3 – 1/2pp. Cambridge, 14 November 1866. 180 x 112 mm. (3) Autograph letter signed to Smith. 3pp. Cambridge, 19 October 1868. 180 x 112 mm. (4) Autograph manuscript (originally enclosed with Cayley’s 19 Oct. 1868 letter). 4ff., tied with linen tape. 412 x 257 mm. N.p., n.d. [1868]. Together 4 items. Very minor marginal tears in nos. (1) and (4), otherwise very good.
Exceptionally rare mathematical correspondence from Arthur Cayley, one of the founders of the British school of pure mathematics, consisting of three autograph letters, all containing mathematics, plus an extensive 4-page mathematical proof written on four extra-large legal sheets. These are the first mathematical letters or manuscripts by Cayley that we have seen on the market in over forty years.
Cayley was the author of over 900 papers covering nearly every aspect of modern mathematics; his greatest contributions were his development of the algebra of matrices, his work in non-Euclidean geometry and n-dimensional geometry, and his contributions to invariant theory. A large number of mathematical constructs bear his name, including Cayley’s theorem (group theory), the Cayley-Hamilton theorem (linear algebra), Cayley’s formula (graph theory) and the Cayley-Klein model (hyperbolic geometry). Cayley’s correspondent was Archibald Smith, who helped to found the Cambridge Mathematical Journal in 1836 and made significant contributions to the study of magnetism and the Earth’s magnetic field.
Both Cayley and Smith were alumni of Trinity College, Cambridge and both subsequently entered Lincoln’s Inn to study law, with Smith being called to the bar in 1841 and Cayley in 1849. Cayley remained in the legal profession until 1863, at which time he left the bar to take the newly established Sadleirean professorship of pure mathematics at Cambridge. It is evident from our letters that Smith and Cayley had a cordial relationship based on their shared love for mathematics; Smith apparently was in the habit of sending Cayley mathematical problems and requesting his help in solving them. Letter (1) contains both Smith’s query and Cayley’s response; in it Smith asked Cayley to give him
"a simple construction for the following problem. Given three points in a plane to draw the straight line such that the sum of the squares of the perpendiculars shall be a minimum."
Cayley responded by writing his solution in the margins of Smith’s letter:
"Considering the points as material points of equal mass, then taking the origin at the C. G. it is easy to show that the required line is the principal axis of least moment. E.g. if the three points be an isosceles base […] less than 60° required line is [parallel] to base—if greater than 60° it is [perpendicular] to base—if equal 60° or triangle is equilateral there the position is indeterminate. The construction for the principal axes depends of course on finding the axes of an elliptic. A. C."
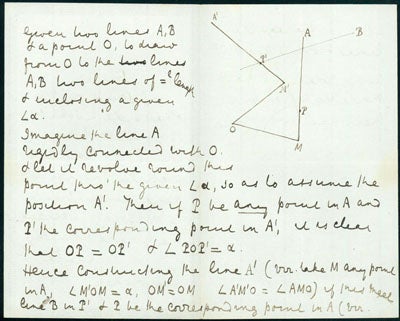
In letter (2), dated 1866, Cayley gave the solution to another of Smith’s mathematical queries; “it comes out easily & prettily enough.” Cayley stated the problem and solution as follows:
"Given two lines A, B & a point O, to draw from O to the lines A, B two lines of [equal] length & enclosing a given [angle] . Imagine the line A rigidly connected with O and let it revolve round this point thro’ the given [angle] , so as to assume the position A’. Then if P be any point in A and P’ the corresponding point in A’, it is clear that OP = OP’ & [angle] POP’ = . . ."
In letter (3), dated 1868, Cayley enclosed the four-page autograph manuscript listed above under no. (4), containing “a solution, such as I have been able to obtain, of your problem, but the solution is I am afraid in a form which will not be of any use to you. May I send the problem—of course in your name—to the Educational Times; it is very likely that you will so obtain a solution of it in a more practical form; and at any rate, the problem, quà problem is an excellent one.” In the manuscript Cayley stated the problem as follows:
"Considering any two circles O, O’ and taking M, M’ the corresponding points in the line OO’, let the angles MP, M’P’ be respectively , – where is a variable angle; it is required to find the envelope of the line PP’."
Cayley’s solution covers four folio pages and includes several equations and two diagrams. Biggs et al., Graph Theory, ch. 3. Dictionary of Scientific Biography. Kline, Mathematical Thought from Ancient to Modern Times, pp. 804-9. Ewald, From Kant to Hilbert, p. 542.
Book Id: 42843Price: $8,500.00